Abstract

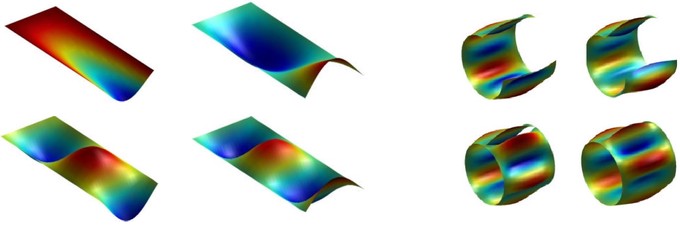
Dynamic stiffness method (DSM) is an alternative to the Finite element method (FEM) in the vibration and buckling analysis. The essential element in the DSM is a continuous element and the corresponding stiffness matrix. The stiffness matrix is formulated based on the exact solution of the governing equations. Consequently, the discretization of the domain is minimized. For a wider application of the DSM, a suitable base of the continuous elements is necessary. Within this thesis, the continuous elements and the corresponding dynamic stiffness matrices for vibration analysis of the Mindlin plate and segment of circular cylindrical shells based on the Donnell-Mushtari and Flugge theory are formulated for the first time. Gorman's method of superposition has been used for solution of the free vibrations problem, while the dynamic stiffness matrix is formulated by using the Projection method. In addition, based on the closed-form solutions of the of free vibration and buckling problem, the following continuous elements are formulated: Maurice Levy plate element based on the Mindlin theory, circular cylindrical shell and segmented circular cylindrical shell with special boundary conditions element based on the Donnell-Mushtari and Flugge theory. The developed stiffness matrices are implemented in the Matlab program for the vibration and buckling analysis of plates and shells assemblies. The results of numerous numerical examples are compared with the available results in the literature, as well as with the results obtained using the FEM, and, in such way, the formulated continuous elements are verified.