Abstract

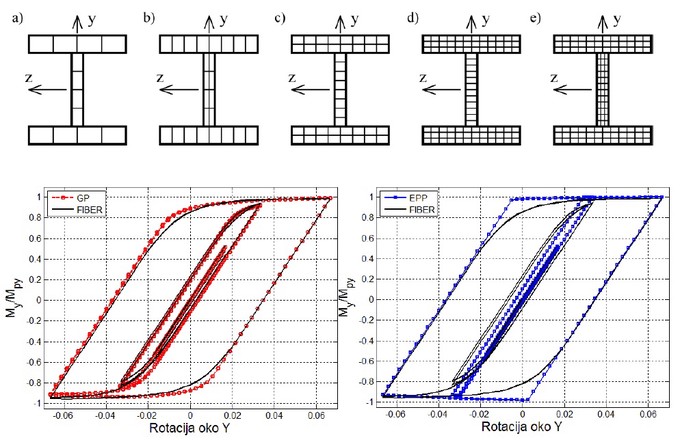
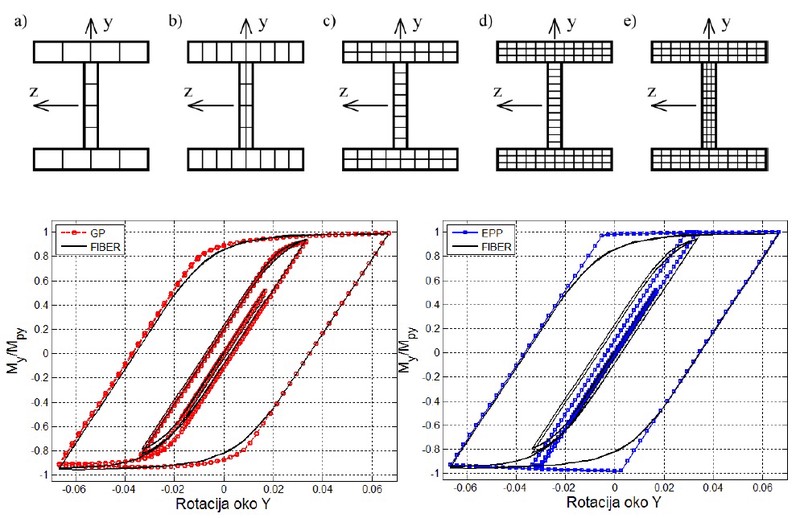
The objective of this study is the efficient nonlinear analysis of space frames with beam/column finite elements considering material nonlinearity. Geometric nonlinearity is taken into account using known methods. Two different nonlinear beam/column elements are analyzed and improved: a fiber element and a concentrated plasticity element. Own computer code is written and implemented into the existing Matlab toolbox for nonlinear structural analysis – FEDEASLab. During this study, the following objectives are achieved. By the optimization of the number and position of material integration points at monitored steel and reinforced concrete cross-sections, the significant improvement in efficiency of the fiber beam-column element under cyclic static and dynamic loading conditions is gained. The practical rules for an efficient discretization of steel wide-flange sections and rectangular reinforced-concrete sections are defined, depending on the desired level of accuracy and the type of analysis. In addition, the new three-dimensional nonlinear beam-column element is formulated. The element is of concentrated plasticity type and overcomes the common limitations of the existing elasto-plastic elements, such as the inability to describe gradual plastification of a cross-section and the hardening behavior. Also, the element keeps its high computational efficiency which is one benefit of concentrated plasticity elements. Two algorithms for element state determination are developed in accordance with the general closest point and convex cutting plane return mapping algorithms. Their influence on element convergence is studied. The ability of the element to simulate frame behavior is confirmed on a number of numerical examples. The special attention is devoted to model CFT composite column behavior. In the study, two different approaches for space frame analysis with beam/column elements are proposed: using fiber element with optimized integration of cross-section and using new developed concentrated plasticity element. Both of these approaches are suitable for use in practice since they ensure results of enough accuracy and are computationally very efficient.