Nonlinear analysis methods are of increasing importance in earthquake-engineering practice with modern performance-based design codes requiring better insight into inelastic structural behavior and more detailed information about the nonlinear structural response than traditional design procedures. Because of the good compromise between accuracy and computational efficiency and the relative ease of response evaluation, frame elements are commonly used in earthquake engineering practice.

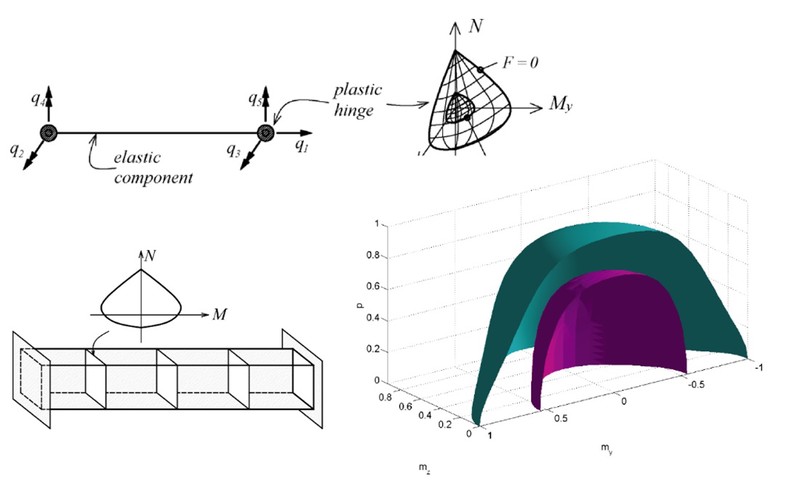
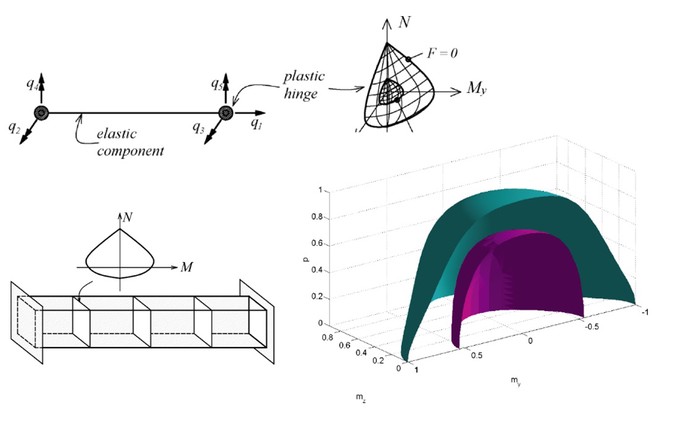
There are two basic types of nonlinear frame elements, the concentrated (lumped) plasticity elements and the distributed inelasticity elements. In the concentrated plasticity elements, the inelastic deformations arise only at the element ends, while the rest of the element behaves elastically. On the other hand, in distributed inelasticity element, inelastic deformations may arise at any of several monitored sections along the element. With either element type, the inelastic behavior of the monitored sections can be described with section resultant relations. The description of the inelastic section behavior with section resultant relations is very common in the simulation of the seismic response of three dimensional structural models. If the resultant constitutive relations arise in the context of a lumped plasticity model, they represent element end force-deformation relations. On the other side, if resultant constitutive relations are used in distributed inelasticity elements, they represent section force-deformation relations.

Work within this topic is focused on the development of new, advanced, 3D frame elements that belong to both of the mentioned two types. The new element of lumped plasticity type is based on the concept of the Generalized plasticity for material stress-strain response put forward by Lubliner, Auricchio and Taylor. The element, denoted as GP, overcomes the most common shortcoming of the elastic-perfectly plastic element response, the absence of gradual yielding and material hardening, while preserving the computational efficiency of elastic-perfectly plastic lumped plasticity beam-column elements. More details about the element formulation and two developed state determination algorithms can be found in paper. The element has proven to be very effective in modeling steel and CFT members. Studies are currently under way to extend the model within the framework of damage plasticity for the representation of the gradual deterioration of strength and stiffness of structural members. Also, formulation of the corresponding GP distributed inelasticity element is under way.